Don’t you wish you could sometimes read people’s minds? Surveys can provide valuable insight into the opinions of a group of people you care about, such as customers, employees, or voters. One of the first steps to creating a survey of any kind is to determine who you will ask to participate. Realistically, it’s unlikely you will be able to get every single person in the group of people you’re interested in to take your survey. You should aim to avoid response bias of people that represent the group as a whole. The participating survey respondents represent what is known in statistics as a sample size.

Figuring out how many people you need to achieve reliable data from your survey involves some detailed mathematics. The guide below offers an easy-to-use sample size calculator to help you determine the appropriate target number for the best results. Additionally, we've listed out key terms and a more in-depth look at how to calculate the complex equation manually. Or you can jump down to our infographic for guidance on How to Create the Best Online Surveys.

{{ CALCULATOR HERE }}

Sample Size Calculator Terms to Know

1. Population Size

The total number of people in the demographic you want to measure. This could be the population of a country, the total number of employees within a company, or the amount of customers who’ve purchased a specific product. It’s OK if you’re unsure of an exact number for your target population, as it’s common to use an estimate for this value.

2. Margin of Error (Confidence Interval)

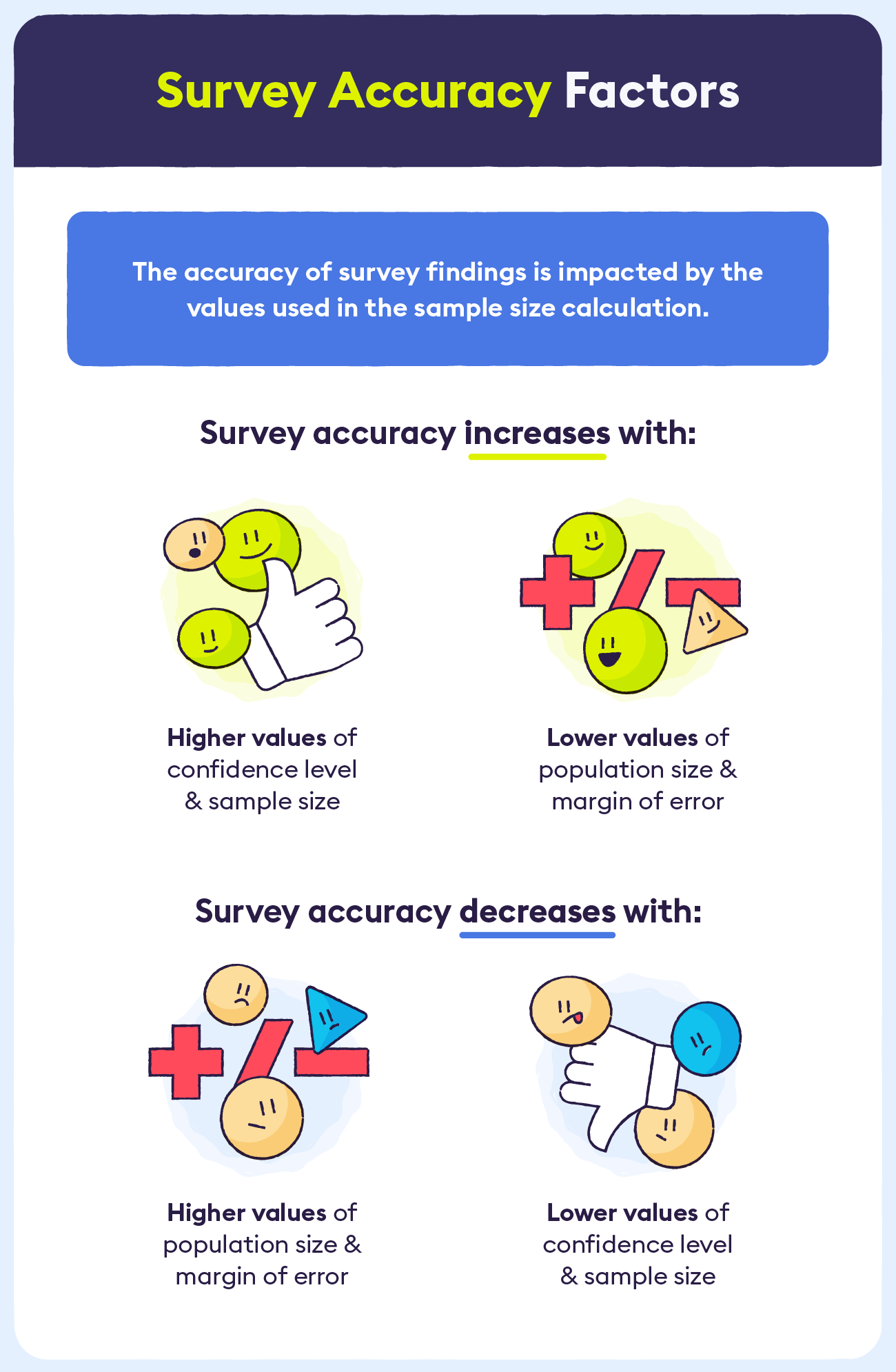
The percentage of error you are willing to allow in your results. It reveals the acceptable proximity of your results to the true value of your target population. It is typically shown as a plus or minus percentage range, such as “+/- 5%.” You’ve probably seen this in practice in news coverage of elections. If you saw that “43% of voters selected Candidate X, with a margin of error of +/- 5%,” the news was reporting their confidence that somewhere between 38% (43 - 5) and 48% (43 + 5) of the entire population of voters made that selection.

3. Confidence Level

The percentage reflecting how sure you are that your results will fall within your margin of error. The most commonly used confidence levels are 90%, 95%, and 99%. Based on our example above, if you select a confidence level of 99%, you are confirming that you are 99% certain your survey data will land in that 38% - 48% we found with our =/- 5% margin of error. The higher you set your confidence level, the greater the accuracy of your end results.

4. Standard Deviation

The estimated percentage of variation you anticipate among your survey results. This number is difficult to calculate prior to running your survey, so best practice is to use 50% (0.5) in your calculations. Sticking with this value will help ensure that your sample size is large enough.

5. Z-score

A constant value determined automatically based on your chosen confidence level. Technically, the z-score represents how many standard deviations are between a selected value and the average number of the population. Also known as a “standard score,” the z-score offers a uniform method of comparing your results to a “normal” population. See Step 2 in the graphic below for a list of the most common z-score values.

How to Calculate Sample Size

Unless you are a fan of math and statistics, manually calculating your sample size may seem a bit arduous. But for those of you curious to understand the factors that will impact your company’s search to find the right amount of survey respondents for your particular needs, we take you step-by-step through the equation below.

To help you make better use of the equation above, here is a longhand example of the mathematical process in real time. Let's calculate the sample size using the inputs below.

Example:

• Population Size (N) = 1000
• Margin of Error (e) = +/- 5% (or 0.05)
• Confidence Level of 95% = z-score (z) = 1.96
• Standard Deviation (p) = 50% (or 0.5)

Based on the formula, the long version of the calculations look like this:

Sample size = [1.962 *0.5(1-0.5)] / 0.052(divided by)1 + [1.962 * 0.5(1-0.5)] / 0.052 * 1000]

Sample size = [3.8416 * 0.25] / 0.0025(divided by)1 + [[3.8416 * 0.25] / 2.5]

Sample size = 384 / 1.384

Sample size (final answer) = 278

In general, it’s widely agreed that a larger sample size leads to more statistically significant results, adding validation to your numbers by showing that there’s less chance that your results happened by chance.

It’s important to be mindful of the risks of aiming for a sample size that is either too large or too small. If you seek out too many participants, your survey can quickly become too expensive and time-consuming to run, with the costs outweighing the benefits. And if you opt for too small of a sample size, you could end up with skewed results due to a disproportionate number of outlier opinions that don’t truly represent your target population.

Impact of Sample Size on Different Types of Surveys

Surveys can serve a multitude of purposes across various industries. And the importance of your sample size may vary according to the goals you establish for each survey. These are several types of surveys to keep in mind based on your needs.

Customer Satisfaction

Measuring perspectives on customer experience can be critical to identifying areas of improvement to benefit your bottom line. For example, surveys are the basis for measuring a company’s net promoter score, which is a metric used by the majority of businesses to gauge and imrpove customer satisfaction. A statistically significant sample size is not critical to these survey results as the content of the feedback has the greater impact.

Employee Satisfaction

Human resources professionals swear by the power of employee satisfaction surveys, as they provide direct input from staff on the internal health of an organization. For this reason, achieving a statistically significant sample size within a company will allow for data applicable to the entire employee population. But even a smaller sample size can be useful in these surveys when quality feedback is the goal.

Political Polls

Surveys on the job approval of political candidates has been a mainstay of election cycles for over fifty years. The results are used for trend purposes to provide insight on the American population’s perception of the country’s well-being. With over 200 million voting-age citizens, a statistically significant sample size of at least 1000 is critical to achieving relevant data.

Market Research

Discovering what your market “thinks” can be a powerful asset in strategizing your business plans. Market research surveys require statistically significant sample sizes in order to ensure the accuracy of the insights tailored to your specific target market.

Take a look at the visual below for additional tips on how to maximize the impact of your results when conducting an online survey.

When you make the choice to conduct a survey, take the time to correctly calculate your sample size and put thought into each element of the survey (even simple demographic questions). If you were to simply take a guess, you could seriously jeopardize the validity of your resulting data—potentially wasting a lot of time and money. Take the guesswork out of the process and let us do the math for you by using the straightforward sample size calculator above.